Specific Heat
The specific heat is the amount of heat per unit mass required to raise thetemperature by one degree Celsius. The relationship between heat and temperature change is usually expressed in the form shown below where c is the specific heat. The relationship does not apply if a phase change is encountered, because the heat added or removed during a phase change does not change the temperature.
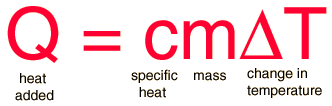
The specific heat of water is 1 calorie/gram °C = 4.186 joule/gram °C which is higher than any other common substance. As a result, water plays a very important role in temperature regulation. The specific heat per gram for water is much higher than that for a metal, as described in the water-metal example. For most purposes, it is more meaningful to compare the molar specific heats of substances.
The molar specific heats of most solids at room temperature and above are nearly constant, in agreement with the Law of Dulong and Petit. At lower temperatures the specific heats drop as quantum processes become significant. The low temperature behavior is described by the Einstein-Debye model of specific heat.
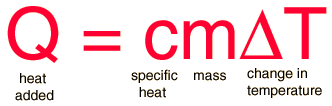
The specific heat of water is 1 calorie/gram °C = 4.186 joule/gram °C which is higher than any other common substance. As a result, water plays a very important role in temperature regulation. The specific heat per gram for water is much higher than that for a metal, as described in the water-metal example. For most purposes, it is more meaningful to compare the molar specific heats of substances.
The molar specific heats of most solids at room temperature and above are nearly constant, in agreement with the Law of Dulong and Petit. At lower temperatures the specific heats drop as quantum processes become significant. The low temperature behavior is described by the Einstein-Debye model of specific heat.
The specific heat (also called specific heat capacity) is the amount of heat required to change a unit mass (or unit quantity, such as mole) of a substance by one degree in temperature. Therefore, unlike the extensive variable heat capacity, which depends on the quantity of material, specific heat is an intensive variable and has units of energy per mass per degree (or energy per number of moles per degree).
The heat capacity of a substance can differ depending on what extensive variables are held constant, with the quantity being held constant usually being denoted with a subscript. For example, the specific heat at constant pressure is commonly denoted  ,
, .
.
| (1) |
i.e., 1 calorie is needed per degree Kelvin (or Celsius) of temperature change for 1 gram of liquid water. In fact, the definition of (one of the several types of) the calorie is the amount of heat needed to change thetemperature of 1 g of water by 1  at its temperature of maximum density (roughly 3.98° C).
at its temperature of maximum density (roughly 3.98° C).
The heat capacity ratio is defined as the ratio of specific heats of a substance at constant pressure and constant volume,
Specific heat is another physical property of matter. All matter has a temperature associated with it. The temperature of matter is a direct measure of the motion of the molecules: The greater the motion the higher the temperature:

Motion requires energy: The more energy matter has the higher temperature it will also have. Typicall this energy is supplied by heat. Heat loss or gain by matter is equivalent energy loss or gain.
With the observation above understood we con now ask the following question: by how much will the temperature of an object increase or decrease by the gain or loss of heat energy? The answer is given by the specific heat (S) of the object. The specific heat of an object is defined in the following way: Take an object of mass m, put in x amount of heat and carefully note the temperature rise, then S is given by

In this definition mass is usually in either grams or kilograms and temperatture is either in kelvin or degres Celcius. Note that the specific heat is "per unit mass". Thus, the specific heat of a gallon of milk is equal to the specific heat of a quart of milk. A related quantity is called the heat capacity (C). of an object. The relation between S and C is C = (mass of obect) x (specific heat of object). A table of some common specific heats and heat capacities is given below:
Consider the specific heat of copper , 0.385 J/g 0C. What this means is that it takes 0.385 Joules of heat to raise 1 gram of copper 1 degree celcius. Thus, if we take 1 gram of copper at 25 0C and add 1 Joule of heat to it, we will find that the temperature of the copper will have risen to 26 0C. We can then ask: How much heat wil it take to raise by 1 0C 2g of copper?. Clearly the answer is 0.385 J for each gram or 2x0.385 J = 0.770 J. What about a pound of copper? A simple way of dealing with different masses of matter is to dtermine the heat capacity C as defined above. Note that C depends upon the size of the object as opposed to S that does not.
We are not in position to do some calculations with S and C.
Example 1: How much energy does it take to raise the temperature of 50 g of copper by 10 0C?

Example 2: If we add 30 J of heat to 10 g of aluminum, by how much will its temperature increase?

Thus, if the initial temperture of the aluminum was 20 0C then after the heat is added the temperature will be 28.3 0C.
Converting between Common Units
Example - Heating Aluminum
2 kg of aluminum is heated from 20 oC to 100 oC. Specific heat of aluminum is 0.91 kJ/kg0C and the heat required can be calculated as
dQ = (2 kg) (0.91 kJ/kg0C) ((100 oC) - (20 oC))
= 145.6 (kJ)
Example - Heating Water
One litre of water is heated from 0 oC to boiling 100 oC. Specific heat of water is 4.19 kJ/kg0C and the heat required can be calculated as
dQ = (1 litre) (1 kg/litre) (4.19 kJ/kg0C) ((100 oC) - (0 oC))
= 419 (kJ)
Specific Heat Gases
There are two definitions of Specific Heat for vapors and gases:
Gas Constant
The gas constant can be expressed as
Ratio of Specific Heat
The Ratio of Specific Heat is expressed
Shailesh kr shukla
directoratace@gmail.com
| ||||||||||||||||||||||||||||||||||||
No comments:
Post a Comment